A more efficient method of the computing complex spatial statistics that geoscientists often face is presented in a new study by researchers from Polytechnique Montréal and the UQ Gas & Energy Transition Research Centre (UQGET).
UQGET Research Fellow Dr Sebastian Hoerning co-authored the paper with Dany Lauzon, and said the method - which is freely available as MATLAB computer code - has a range of applications.
“Nature produces rather complex spatial structures. Geostatistics, a part of statistics that deals with spatial data, tries to describe and model these structures. Often this involves a simplifying assumption – symmetry. The world however is rarely symmetric,” said Dr Hoerning.
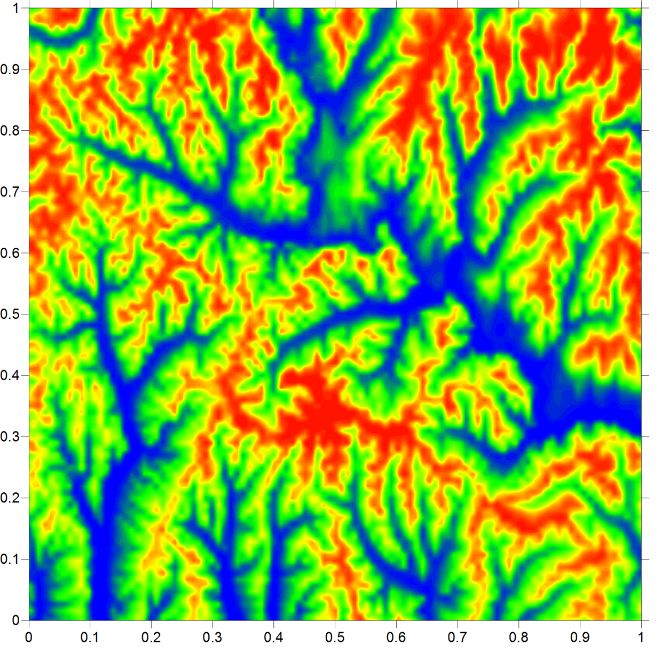
“Let’s take topography as an example. Figure 1 shows a normalized topographical map without any information on the actual elevations or the location. If the world was symmetric, mountains and valleys would look the same in terms of their topographical patterns. This is obviously not the case and the asymmetry, i.e., the difference in the spatial structures enables us to tell what the mountains are and what the valleys are in this figure.”
“To geostatistically describe this asymmetry and other features of these spatial structures can be computationally very expensive. Therefore, our recent publication introduces an approach to very efficiently calculate these geostatistical descriptors which makes use of an established algorithm that has the efficiency as part of its name - fast Fourier transform. The approach has many potential applications, for example, in geology, hydrology, or meteorology.”



